Creative bathroom tiling isn’t just a problem for DIY home renovators! It is also one of the hardest and most interesting problems in mathematics. Imagine a bathroom floor made up of black and white tiles. They are the same shape and fit together perfectly, so no overlaps or gaps. You are probably imagining rectangles or hexagons, something you might even have yourself, a ‘periodic’ pattern that will repeat forever. So is it possible to find an ‘aperiodic’ shape that will not repeat? Even if your bathroom went on forever?
This question transfixed mathematicians for about 50 years, with mathematician Robert Berger even stating in 1966 it was ‘probably unprovable,’ with several other mathematicians giving up hope.
Until now, aperiodic tiling required at least two tiles of different shapes. Robert Berger originally found a massive set of 20,426 differently coloured tiles, which must have taken a while. Thankfully, he reduced his set of never-repeating tiles to a slightly more palatable set of 104. In the decades to come, mathematicians brought the number of tiles in an aperiodic set down.
In 1968 a computer scientist named Donald Knuth found an example with 92. Three years later mathematician Raphael Robinson found a variant with only six tile types. Finally, in 1974, Roger Penrose presented his solution using only two tiles. It is absolutely beautiful and has since become an iconic tiling pattern.

However, progress on the problem started to stall. For years mathematicians tried to find the elusive ‘Einstein’ tile — not a name in tribute of the famous physicist, but because in German it literally means ‘one stone’, or rather one tile. Even Roger Penrose eventually gave up!
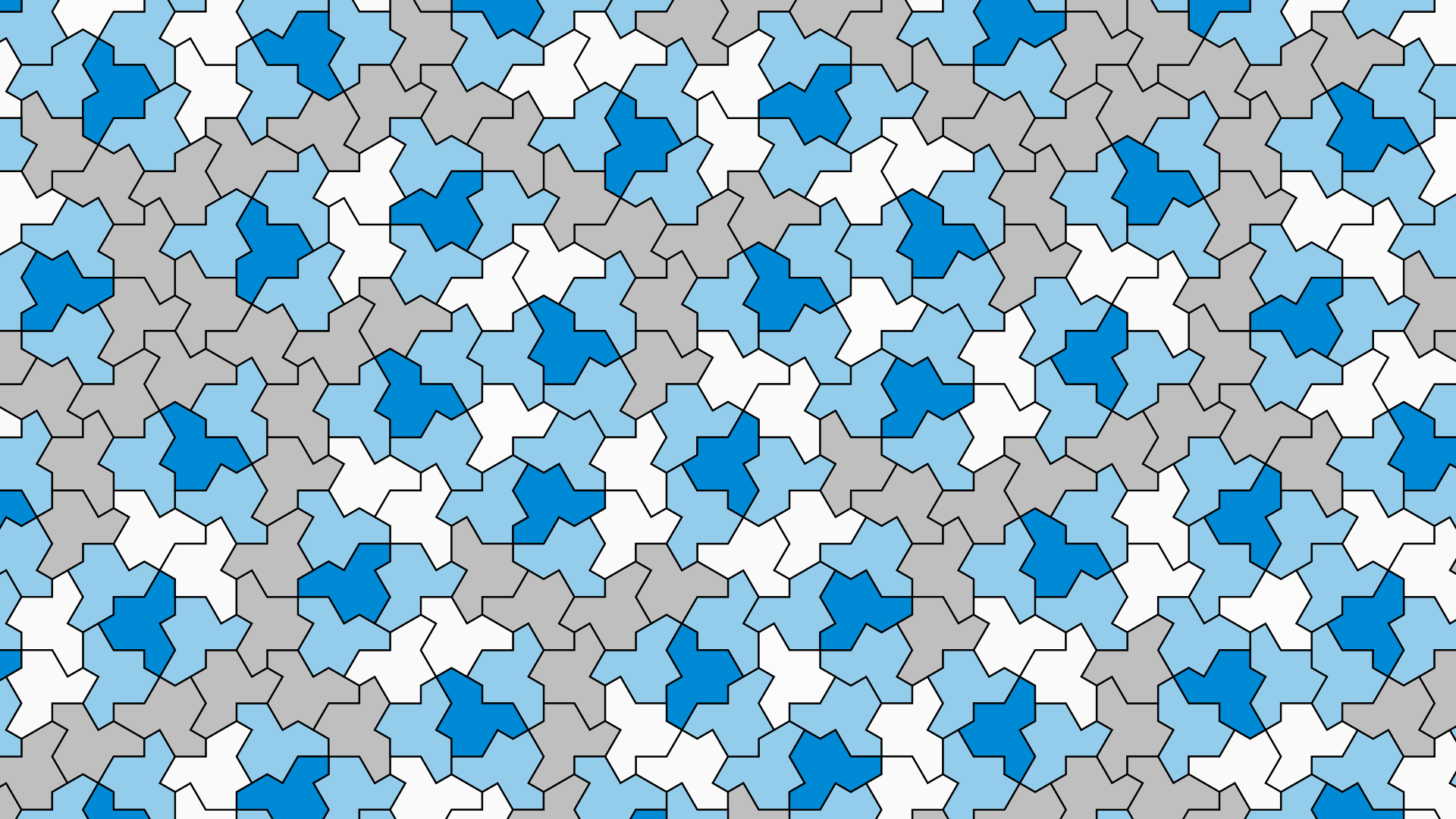
This makes what actually happened quite extraordinary. In November 2022, retired printing system engineer and puzzles enthusiast 64-year-old David Smith from Yorkshire was playing with patterns and found a rough 13-sided shape that can be made from 8 congruent kites he believed to be the elusive Einstein. He called the now-famous shape a ‘hat’. With the help of software, he lined up more and more tiles. It looked like they might be able to cover the plane. However, a repeating pattern could emerge.
He called Canadian Craig Kaplan, a computer scientist at the University of Waterloo in Ontario, who instantly saw the potential of the shape. Along with a software developer Joseph Samuel Myers and a mathematician with extensive tiling experience, Chaim Goodman-Strauss from the University of Arkansas, Kaplan proved that Smith’s singular ‘monotile’ indeed paves the plane without gaps nor repetition. They were amazed at the simplicity of the 13-sided shape, with Goodman-Strauss saying “I would’ve drawn some crazy, squiggly, nasty thing,” to Science News.
Even more amazingly, they found that Smith had discovered not only one but an infinite number of Einstein tiles! This is because there were two entirely different, and even simpler shapes which are also aperiodic, and as one transforms to the other it passes through an infinite number of shapes which are also, to give them the fancy term, ‘aperiodic monotiles’.
This is a great example of some of the funny and largely irrelevant puzzles mathematicians can spend extraordinary amounts of time studying. Often, they are unproven or unprovable and you never hear of it again, or, like the Einstein problem eventually solved by pure determination. And, as it seems, a retired puzzle buff from Yorkshire!
It might not be wise for you try to solve these, but it’s fun to learn about this sort of conundrum. However, If you are still interested, It might be wise to visit Scientific American’s detailed article about the Einstein to find out more. And if you would like to play with Einsteins, Penrose Tiles and even ‘Nature’ tiles to create a mosaic that will never repeat with Mathigon’s Polypad mathematical playground.
It all goes to show that tiling a bathroom can be made a little more complex if you put your mind to it!